MATH 101 Sample Mastery Exam
MULTIPLE CHOICE
- The set of elements in {x | x is a positive even integer less than 4} is:
- {1, 2, 3,}
- {2}
- {0, 2}
- {..., -2, 0, 2}
- None of these
- If U = {a, b, c, d, e}, A = {a, c, e} and B = {a, b, e}, then (A
 B) =
B) =
- {b, c, d}
- {b}
- {a, e}
- {a, c, d, e}
- None of these
- If U, A and B are as in the previous problem and C = {b, c, d}. Then (A'
 B)
B)  C =
C =
- U
- {b, e}
- {a, b, d, e}
- {}
- None of these
- In the figure, the numbered regions that identify the set (A
 B)
B)  C are:
C are:
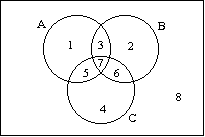
- 1, 2, 3
- 3, 4, 5, 6, 7
- 3, 7
- 5, 6, 7
- None of these
- In Roman numerals, 1982 is
- I IX VIII II
- MCMLXXXII
- CMMXXXLII
- IXX VIII II
- None of these
- In decimal notation, the Roman numeral XLVIII is
- 1058
- 10,508
- 44
- 68
- 48
- Written in decimal notation, (5 x 103) + (3 x 102) + (9 x 100) =
- 5390
- 5039
- 5309
- 539
- None of these
- Written as a decimal, 3.67% is
- 0.367
- 0.00367
- 0.0367
- 3.67
- None of these
- Written as a percent, correct to one decimal place, 2/3 is
- 0.6%
- 66.7%
- 67%
- 66.6%
- None of these
- About 4 million women make between $25,000 and $50,000 annually. If this represents 14.5% of all working women, about how many working women are there?
- 276 million
- 28 million
- 58 million
- 145 million
- None of these
- The hypotenuse of a right triangle is 8 ft longer than one leg and 4 ft longer than the other leg. What are the dimensions of this triangle?
- 2 ft by 6 ft by 10 ft
- 4 ft by 8 ft by 12 ft
- 9 ft by 13 ft by 17 ft
- 12 ft by 16 ft by 20 ft
- None of these
- Suppose you rent a car for one day at the rate of $12 per day plus 10 cents per mile. How many miles could you drive for a rental charge of $45?
- 33
- 330
- 350
- 450
- None of these
- The cost of gasoline per hr for running a certain car is directly proportional to the square of the speed. If the cost is $2.40 per hr for a speed of 40 mph, what is the cost per hr for a speed of 60 mph?
- $3.60
- $4.80
- $5.00
- $5.40
- None of these
- The graph of the function defined by g(x) = x2 - 2, x an integer, and -2 ≤ x ≤ 2 is
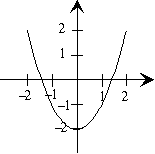
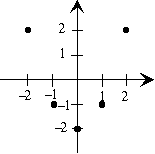
- None of these
- Which of the following relations are functions?
- {(x, y) | y = x2 + 2}
- {(x, y) | y2 = x + 2}
- {(2, 1), (3, 1), (4, 1)}
- i only
- ii only
- iii only
- i and iii only
- None of these
- A window is in the shape of a rectangle surmounted by a semicircle. The width of the window is 4 ft, which is also the diameter of the circle, and the height of the rectangular part is 4 ft. Find the total area of this window.
- (16 + 16
 ) ft2
) ft2
- (16 + 4
 ) ft2
) ft2
- (16 + 2
 ) ft2
) ft2
- (16 + 8
 ) ft2
) ft2
- None of these
- A circle of diameter 6 in. has its center at the center of a square of side 6 in. Find the area of the region that is inside the square and outside the circle.
- (36
 - 36) in2
- 36) in2
- (36 - 6
 ) in2
) in2
- (36- 9
 ) in2
) in2
- (12
 - 36) in2
- 36) in2
- None of these
- The dimensions of a rectangular box are 3 ft by 3 ft by 5 ft. Find the total surface area of this box.
- 70 ft2
- 78 ft2
- 45 ft2
- 120 ft2
- None of these
- A restaurant offers a choice of 2 soups, 4 entrees, and 3 desserts. The number of different possible meals consisting of a soup, an entree, and a dessert is:
- 10
- 20
- 24
- 30
- None of these
- Suppose that one of two dice is white and the other is black. If the result of a toss is distinguished by both numbers and colors, how many different results are possible?
- 72
- 36
- 24
- 12
- None of these
- How many distinct arrangements (in a row) can be made with the letters in the word STAMP?
- 720
- 120
- 24
- C(5, 5)
- None of these
- Johnny has 4 coins: a penny, a nickel, and two dimes. How many different sums of money can Johnny form by using 3 of these coins?
- C(4, 3)
- 3
- 4
- 6
- None of these
- A single fair die is rolled. The probability of obtaining a number other than 4 or 5 is
- 1/6
- 1/3
- 2/3
- 5/6
- None of these
- Two cards are drawn at random and without replacement from a standard deck of 52 cards. The probability that one card is red and the other is black is
- 13/51
- 26/51
- 1/26
- C(26, 2)/C(52, 2)
- None of these
- If two cards are drawn as in the previous problem, the probability that neither card is a king or a queen is
- 13/51
- 26/51
- 1/26
- C(4, 2)/C(52, 2)
- None of these
- A single fair die is rolled. The probability of obtaining a number greater than 4 is
- 1/6
- 1/3
- 2/3
- 5/6
- None of these
- A fair coin is tossed 6 times. The probability of getting at least one head is
- 1/2
- 3/4
- 31/32
- 63/64
- None of these
During a certain week in the winter, the following minimum temperatures (all in degrees F) were recorded in an eastern city: 20,31, 25, 32, 26, 36, 33. These data are to be used in problems 28-32.
- The mean of these temperatures is
- 32
- 31
- 29
- 28
- None of these
- The mode of these temperatures is
- 32
- 31
- 29
- 28
- There is none
- The median of these temperatures is
- 32
- 31
- 29
- 28
- None of these
- The range of these temperatures is
- 4 degrees
- 8 degrees
- 16 degrees
- 20 degrees
- 25 degrees
- The standard deviation of these temperatures is
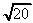
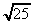
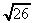
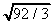
- None of these
- A fair coin is tossed 144 times and the number of heads is recorded. If this experiment is repeated many times, the number of heads will form an approximately normal distribution with a mean of 72 and a standard deviation of 6. Within what limits should the number of heads in 144 tosses be expected to lie?
- 45 and 55
- 54 and 90
- 50 and 100
- 40 and 80
- None of these
- For the coin tossing experiment of the previous problem, what is the probability that heads will occur fewer than 60 times in 144 tosses?
- 0.025
- 0.135
- 0.16
- 0.5
- None of these
- The scores on a multiple-choice test taken by 2000 students were normally distributed with a mean of 60 and a standard deviation of 6. The z-score corresponding to a test score of 75 is
- 1
- 1.5
- 2
- 2.5
- None of these

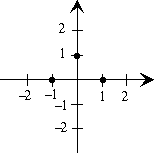
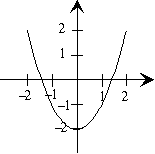


 ) ft2
) ft2 ) ft2
) ft2  ) ft2
) ft2  ) ft2
) ft2  - 36) in2
- 36) in2  ) in2
) in2  ) in2
) in2  - 36) in2
- 36) in2